Numerical models of thermally-driven dynamos: the geodynamo
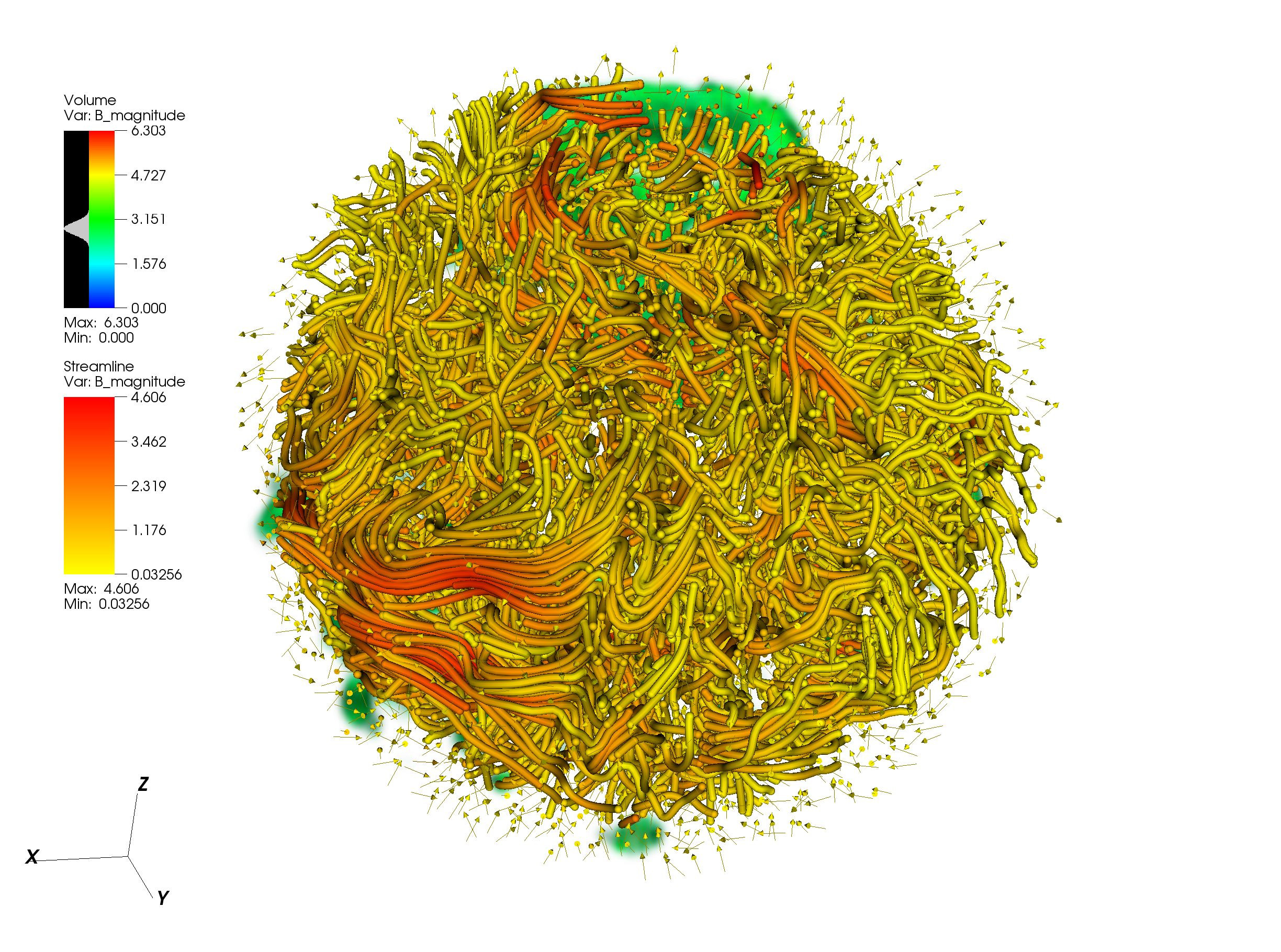
Streamlines of the magnetic field produced in the model
Tangent to the magnetic field lines. Side-view. The z-axis indicates the axis of rotation. Green background is a volume rendering of the magnetic field. Arrows illustrate direction of the surface magnetic field. Magnetic field lines are strongly twisted producing significant amounts of radial field. This radial field is observed on the core-mantle boundary as strong field patches. (http://dx.doi.org/10.3929/ethz-a-010198511, Case 1)
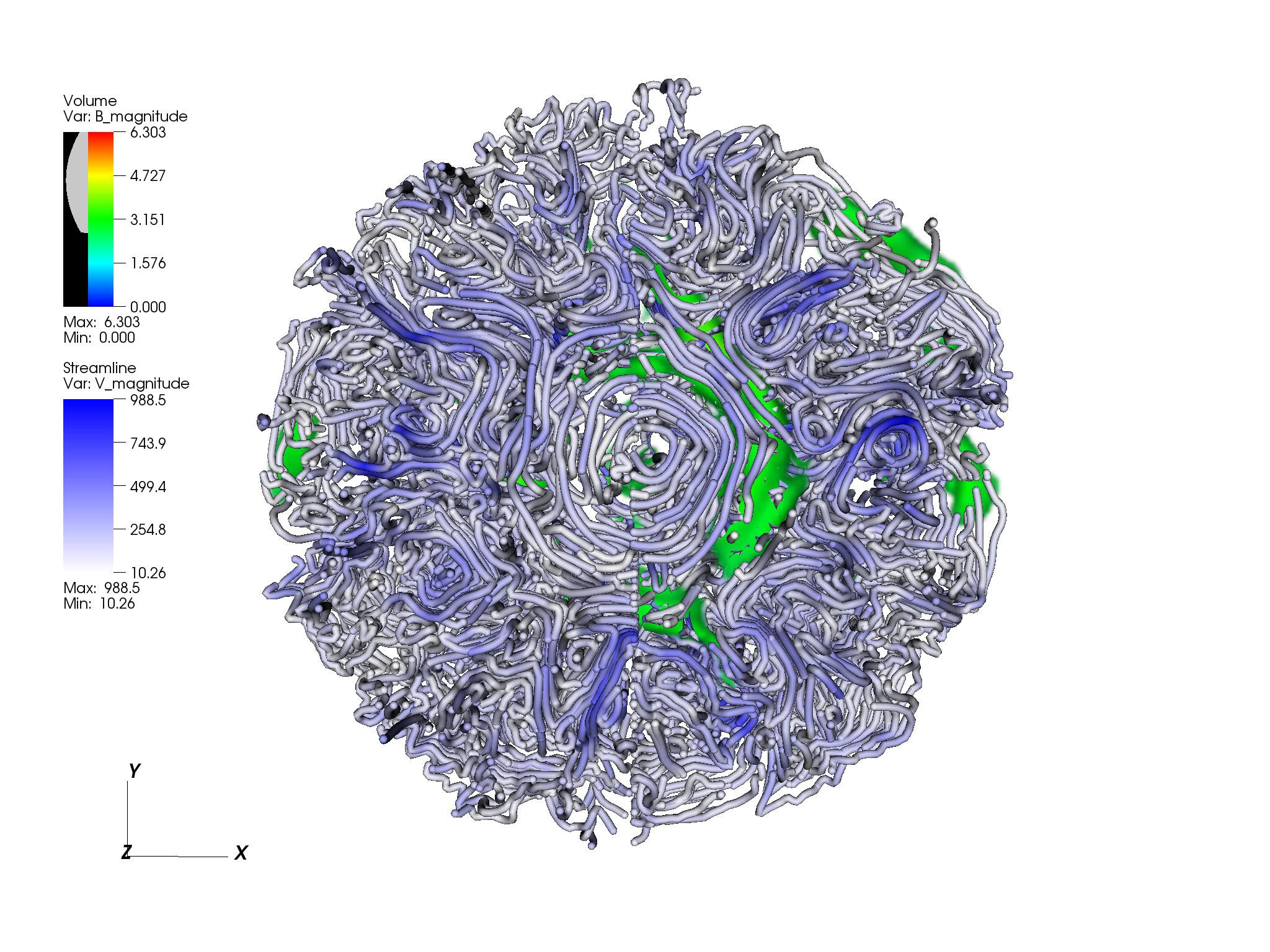
Streamlines of the flow of the liquid metal in the model.
Motions of the liquid metal inside the Earth's outer core are responsible for the large scale magnetic fields observable on our planet. In these numerical experiments a conducting incompressible fluid is enclosed inside of a rotating spherical shell. Energy driving the convection is provided both from the inner boundary (from the slowly growing hot inner core) and through the volumetrically distributed sources (e.g. from the radioactive decay of unstable isotopes). Parameters (rotation rate; electrical, thermal and mechanical properties of the fluid) are chosen in computational limits to be relevant to the Earth's core. Magnetic fields obtained numerically are self-maintained. The core-mantle boundary pattern of the computed magnetic field mimics important features of the observable geomagnetic field.
View from the North Pole. The z-axis indicates the axis of rotation. Green background is a volume rendering of the magnetic field. The central vortex around the rotational axis forms circular-shaped streamlines. One can see the event when the vortex drifts out its central position towards the periphery of the spherical shell. The tip of the vortex is associated with the strong magnetic field observable on the core-mantle boundary. In the Earth's core such an event would be observableon the core-mantle boundary as a displacement of a strong magnetic field patch from the polar region towards lower latitudes.
Low-viscous simulation of the geodynamo.
A vortex containing strong magnetic field is formed on the axis of rotation. We see the event when the vortex becomes unstable and moves towards periphery of the spherical shell.
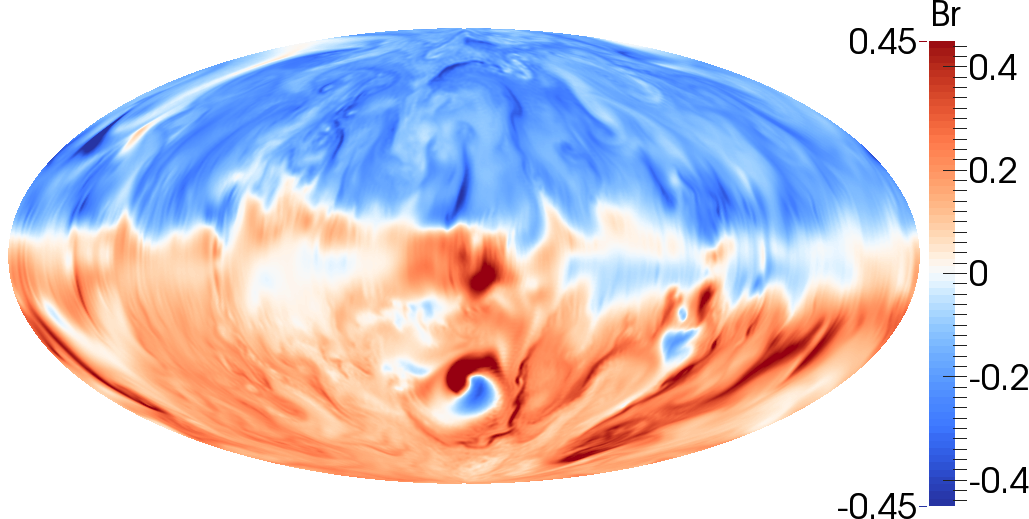
Top: Magnetic field on the core-mantle boundary; Bottom: fields in the middle of the core above equatorial plane. Bottom left: Magnetic field. Bottom right: Temperature field.